Number of Tangents from a Point on a Circle
In this section let us see the relationship betwween a point and tangents from the point to the circle. The point can either be inside, on (or) outside the circle. Let us look at each case one by one.
Case 1: P lies inside the circle
Can you draw a tangent to the circle through this point P? Try to draw a line through the point P. Make sure you extend the line on both sides.
Conclusion: So, it is not possible to draw any tangent to a circle through a point inside it.
Case 2: P lies on the circle
Can you draw a tangent to the circle through this point P?
Observation:
Conclusion: Thus,there is only one tangent to the circle at a point located on the circle.
Case 3: P lies outside the circle
Can you draw a tangent to the circle through this point P?
Observation:
Conclusion: So, exactly two tangents can be drawn to a circle through a point located outside the circle.
Let us consider the third case where T1 and T2 are the points of contact of the tangents PT1 and PT2 respectively.
The length of the segment of the tangent from the external point P and the point of contact with the circle is called the length of the tangent from the point P to the circle.
What can we conclude about PT1 and PT2? As it turns out both
Can we prove that?
Length of tangents from an external point
Theorem:The lengths of tangents drawn from an external point to a circle are equal.
Let's apply the above concepts and check which one has to be applied.
Proof: Given, circle with centre O has a point P outside the circle.
We draw two tangents PR and PQ from the point. We need to prove that PQ = PR
Construction: Join radii OR and OQ. Also join OP. This gives us △OQP and △ORP.
In △OQP and △ORP:
(1) OQ =
(2) OP =
(3) ∠OQP = ∠
Thus, by
△OQP ≅ △
By CPCT:
Hence, the lengths of tangents drawn from an external point to a circle are
Remarks :
- The theorem can also be proved by using the Pythagoras Theorem as follows:
- Note also that ∠ OPQ = ∠ OPR. Therefore, OP is the angle bisector of ∠ QPR, i.e., the centre lies on the bisector of the angle between the two tangents.
1. Prove that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
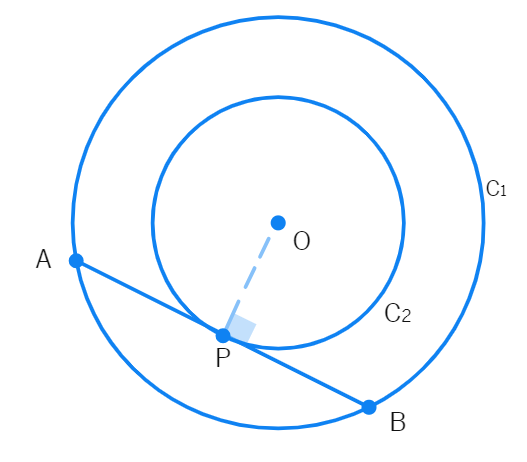
- Let's join OP.Now, AB is a tangent to C2 at P and OP is its radius.
- Now, we know that OP is
to AB. - We also see that AB is a
of the circle C1. - Therefore, OP is the bisector of the chord AB, as the perpendicular from the centre bisects the chord.
- Hence, AP =
.
2. Two tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ∠ PTQ = 2 ∠OPQ.
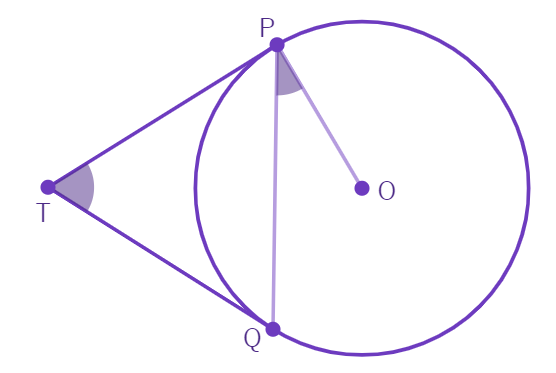
- Let ∠ PTQ = θ. We also know that TP =
. Thus, △TPQ is an triangle - ∠ TPQ = ∠
= 1 2 180 ° − θ ° - θ 2 - We also know ∠ OPT =
° - ∠ OPQ = ∠
– ∠ = 90° – ( 90 ° − θ 2 - Hence, ∠ PTQ = 2 ∠ OPQ.
3. PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T. Find the length TP.
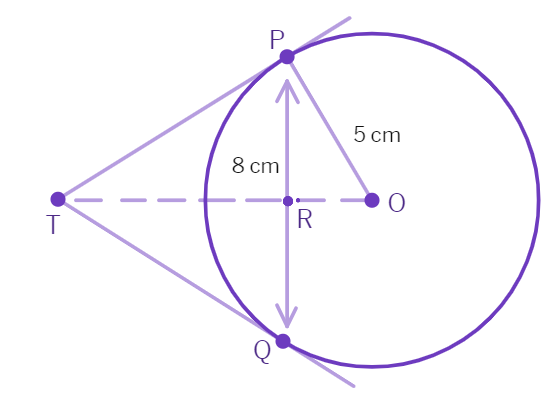
- Join OT and let it intersect PQ at the point R. Also Δ TPQ is
and TO is the angle bisector of ∠ - Thus, OT ⊥
and OT bisects PQ which gives PR = RQ = cm. - OR =
OP 2 − PR 2 5 2 − 4 2 cm. - Now, ∠ TPR + ∠ RPO =
° = ∠ TPR + ∠ PTR - ∠ RPO = ∠
- Therefore, Δ TRP is similar to the Δ PRO by AA similarity. This gives:
TP PO - Putting values: TP =
cm. - Calculating
- Value has been found.
Alternate Method: TP can also be found by using the Pythagoras Theorem, as follows:
Let TP = x and TR = y. Then
Subtracting (1) from (2), we get
y =
=
or x =
Prove that the tangents drawn at the ends of a diameter of a circle are parallel.
First, draw a circle and connect two points, A and B, such that AB becomes the diameter of the circle. Now, draw two tangents, PQ and RS, at points A and B, respectively.
Now, both radii, i.e. AO and OB, are perpendicular to the tangents.
So, OB is perpendicular to RS, and OA is perpendicular to PQ.
So, ∠OAP = ∠OAQ = ∠OBR = ∠OBS = 90°
From the above figure, angles OBR and OAQ are alternate interior angles.
Also, ∠OBR = ∠OAQ and ∠OBS = ∠OAP (Since they are also alternate interior angles)
So, it can be said that line PQ and line RS will be parallel to each other (Hence Proved).
Prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
Let, O is the centre of the given circle.
A tangent PR has been drawn touching the circle at point P.
Draw QP ⊥ RP at point P, such that point Q lies on the circle.
Circle with Tangent PR
∠OPR = 90° (radius ⊥ tangent)
Also, ∠QPR = 90° (Given)
∴ ∠OPR = ∠QPR
Now, the above case is possible only when centre O lies on the line QP.
Hence, perpendicular at the point of contact to the tangent to a circle passes through the centre of the circle.
The length of a tangent from a point A at distance 5 cm from the centre of the circle is 4cm. Find the radius of the circle.
Here, AB is the tangent that is drawn on the circle from point A.
So, the radius OB will be perpendicular to AB, i.e., OB ⊥ AB
We know, OA = 5cm and AB = 4 cm
Now, In △ABO,
OA2 =AB2+BO2 (Using Pythagoras’ theorem)
52 = 42+BO2
BO2 = 25-16
BO2 = 9
BO = 3
So, the radius of the given circle, i.e., BO, is 3 cm.
Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
Draw two concentric circles with the centre O. Now, draw a chord AB in the larger circle, which touches the smaller circle at a point P, as shown in the figure below.
Ncert solutions class 10 chapter 10-9
From the above diagram, AB is tangent to the smaller circle to point P.
∴ OP ⊥ AB
Using Pythagoras’ theorem in triangle OPA,
OA2= AP2+OP2
52 = AP2+32
AP2 = 25-9
AP = 4
Now, as OP ⊥ AB,
Since the perpendicular from the centre of the circle bisects the chord, AP will be equal to PB.
So, AB = 2AP = 2×4 = 8 cm
So, the length of the chord of the larger circle is 8 cm.
A quadrilateral ABCD is drawn to circumscribe a circle (see Fig. 10.12). Prove that AB + CD = AD + BC.
The figure given is:
Ncert solutions class 10 chapter 10-10
From the figure, we can conclude a few points, which are
(i) DR = DS
(ii) BP = BQ
(iii) AP = AS
(iv) CR = CQ
Since they are tangents on the circle from points D, B, A, and C, respectively.
Now, adding the LHS and RHS of the above equations, we get,
DR+BP+AP+CR = DS+BQ+AS+CQ
By rearranging them, we get,
(DR+CR) + (BP+AP) = (CQ+BQ) + (DS+AS)
By simplifying,
AD+BC= CD+AB
In Fig. 10.13, XY and XY are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting XY at A and XY at B. Prove that AOB = 90°.
From the figure given in the textbook, join OC. Now, the diagram will be as:
Now, the triangles △OPA and △OCA are similar using SSS congruency as
(i) OP = OC They are the radii of the same circle
(ii) AO = AO It is the common side
(iii) AP = AC These are the tangents from point A
So, △OPA ≅ △OCA
Similarly,
△OQB ≅ △OCB
So,
∠POA = ∠COA … (Equation i)
And, ∠QOB = ∠COB … (Equation ii)
Since the line POQ is a straight line, it can be considered as the diameter of the circle.
So, ∠POA +∠COA +∠COB +∠QOB = 180°
Now, from equations (i) and equation (ii), we get,
2∠COA+2∠COB = 180°
∠COA+∠COB = 90°
∴ ∠AOB = 90°
Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the centre.
First, draw a circle with centre O. Choose an external point P and draw two tangents, PA and PB, at point A and point B, respectively. Now, join A and B to make AB in a way that subtends ∠AOB at the centre of the circle. The diagram is as follows:
Ncert solutions class 10 chapter 10-12
From the above diagram, it is seen that the line segments OA and PA are perpendicular.
So, ∠OAP = 90°
In a similar way, the line segments OB ⊥ PB and so, ∠OBP = 90°
Now, in the quadrilateral OAPB,
∴ ∠APB+∠OAP +∠PBO +∠BOA = 360° (since the sum of all interior angles will be 360°)
By putting the values, we get,
∠APB + 180° + ∠BOA = 360°
So, ∠APB + ∠BOA = 180° (Hence proved).
Prove that the parallelogram circumscribing a circle is a rhombus.
Consider a parallelogram ABCD which is circumscribing a circle with a centre O. Now, since ABCD is a parallelogram, AB = CD and BC = AD.
Ncert solutions class 10 chapter 10-13
From the above figure, it is seen that,
(i) DR = DS
(ii) BP = BQ
(iii) CR = CQ
(iv) AP = AS
These are the tangents to the circle at D, B, C, and A, respectively.
Adding all these, we get
DR+BP+CR+AP = DS+BQ+CQ+AS
By rearranging them, we get
(BP+AP)+(DR+CR) = (CQ+BQ)+(DS+AS)
Again by rearranging them, we get
AB+CD = BC+AD
Now, since AB = CD and BC = AD, the above equation becomes
2AB = 2BC
∴ AB = BC
Since AB = BC = CD = DA, it can be said that ABCD is a rhombus.
A triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 8 cm and 6 cm respectively (see Fig. 10.14). Find the sides AB and AC.
Consider the triangle ABC,
We know that the length of any two tangents which are drawn from the same point to the circle is equal.
So,
(i) CF = CD = 6 cm
(ii) BE = BD = 8 cm
(iii) AE = AF = x
Now, it can be observed that,
(i) AB = EB+AE = 8+x
(ii) CA = CF+FA = 6+x
(iii) BC = DC+BD = 6+8 = 14
Now the semi-perimeter “s” will be calculated as follows
2s = AB+CA+BC
By putting the respective values, we get,
2s = 28+2x
s = 14+x
Ncert solutions class 10 chapter 10-15
By solving this, we get,
= √(14+x)48x ……… (i)
Again, the area of △ABC = 2 × area of (△AOF + △COD + △DOB)
= 2×((½×OF×AF)+(½×CD×OD)+(½×DB×OD))
= 2×½(4x+24+32) = 56+4x …………..(ii)
Now from (i) and (ii), we get,
√(14+x)48x = 56+4x
Now, square both sides,
48x(14+x) = (56+4x)2
48x = (4(14+x))2/(14+x)
48x = 16(14+x)
48x = 224+16x
32x = 224
x = 7 cm
So, AB = 8+x
i.e. AB = 15 cm
And, CA = x+6 =13 cm.
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
First, draw a quadrilateral ABCD which will circumscribe a circle with its centre O in a way that it touches the circle at points P, Q, R, and S. Now, after joining the vertices of ABCD, we get the following figure:
Ncert solutions class 10 chapter 10-16
Now, consider the triangles OAP and OAS.
AP = AS (They are the tangents from the same point A)
OA = OA (It is the common side)
OP = OS (They are the radii of the circle)
So, by SSS congruency △OAP ≅ △OAS
So, ∠POA = ∠AOS
Which implies that∠1 = ∠8
Similarly, other angles will be
∠4 = ∠5
∠2 = ∠3
∠6 = ∠7
Now by adding these angles, we get
∠1+∠2+∠3 +∠4 +∠5+∠6+∠7+∠8 = 360°
Now by rearranging,
(∠1+∠8)+(∠2+∠3)+(∠4+∠5)+(∠6+∠7) = 360°
2∠1+2∠2+2∠5+2∠6 = 360°
Taking 2 as common and solving, we get
(∠1+∠2)+(∠5+∠6) = 180°
Thus, ∠AOB+∠COD = 180°
Similarly, it can be proved that ∠BOC+∠DOA = 180°
Therefore, the opposite sides of any quadrilateral which is circumscribing a given circle will subtend supplementary angles at the centre of the circle.
