Exercise 8.1
1. If the diagonals of a parallelogram are equal, then show that it is a rectangle.
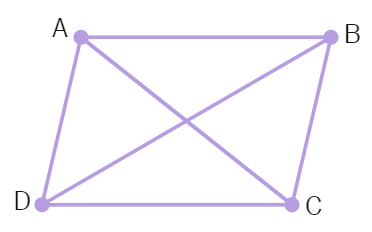
2. Show that the diagonals of a square are equal and bisect each other at right angles.
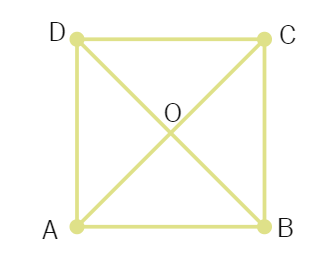
3. Diagonal AC of a parallelogram ABCD bisects ∠ A. Show that:
(i) it bisects ∠ C also,
(ii) ABCD is a rhombus.
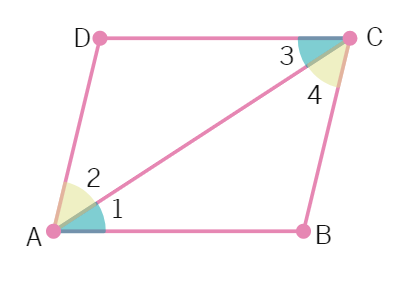
thus, AB =
4. ABCD is a rectangle in which diagonal AC bisects ∠ A as well as ∠ C. Show that:
(i) ABCD is a square
(ii) diagonal BD bisects ∠ B as well as ∠ D.
Solution:
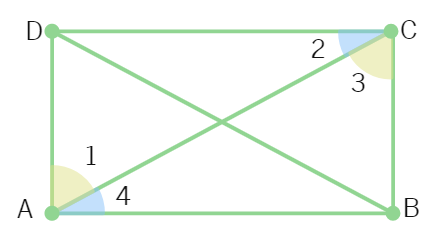
5. In parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ. Show that:
(i) ∆ APD ≅ ∆ CQB
(ii) AP = CQ
(iii) ∆ AQB ≅ ∆CPD
(iv) AQ = CP
(v) APCQ is a parallelogram
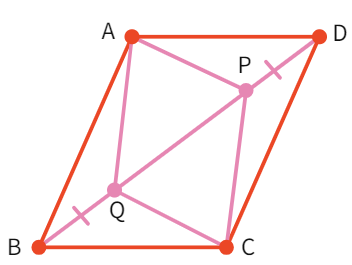
- Consider the triangles ΔAPD and ΔCQB: DP =
- ∠ADP = ∠
(Alternate interior angles) - AD =
(Opposite sides of a parallelogram) - By
congruency rule, ΔAPD ≅ Δ - By CPCT, AP =
- Now, considering the triangles ΔAQB and ΔCPD:
- BQ =
and ∠ABQ = ∠ (Alternate interior angles) - Also,
= CD (Opposite sides of a parallelogram) - Thus, by
congruency, ΔAQB ≅ Δ - By CPCT, AQ =
- For quadrilateral APCQ, we have proved that the opposite sides are equal: AQ = CP and AP = CQ
- Thus, APCQ is a parallelogram.
6. ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD. Show that:
(i) ∆ APB ≅ ∆ CQD
(ii) AP = CQ
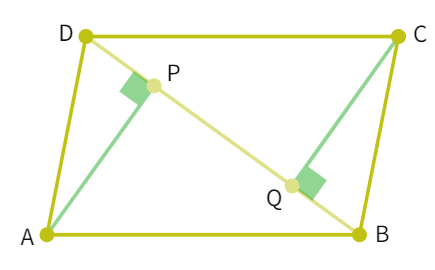
- Considering the triangles ΔAPB and ΔCQD: ∠ABP = ∠
(Alternate interior angles) - ∠APB = ∠
(= 90° as AP and CQ are perpendiculars) - AB =
(opposite sides of parallelogram) - By
congruency rule: ΔAPB ≅ Δ - By CPCT: AP =
- Hence, proved.
7. ABCD is a trapezium in which AB || CD and AD = BC. Show that:
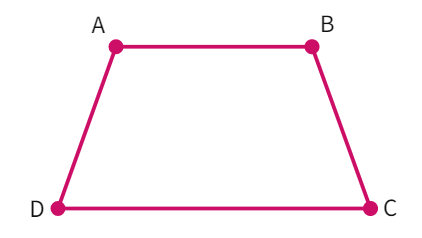
(i) ∠ A = ∠ B
(ii) ∠ C = ∠ D
(iii) ∆ ABC ≅ ∆ BAD
(iv) diagonal AC = diagonal BD
Before starting the proof, let's construct a line through C parallel to DA intersecting AB produced at E (as shown in the below figure). We obtain a parallelogram AECD.
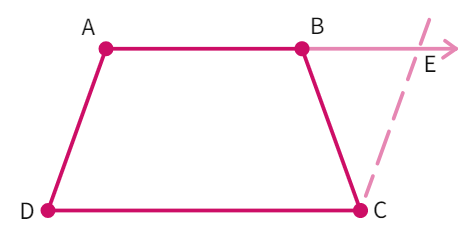
- Considering the triangle BCE: CE =
(Opposite sides of a parallelogram) (i) - From (i) and (ii), BC =
i.e. the triangle BCE is an triangle. - This means ∠CBE = ∠
(Angles opposite to equal sides) - We also have: ∠A + ∠CEB =
° (Angles on the same side of transversal) - We can re-write this as: ∠A + ∠
= 180° - We also have: ∠B + ∠CBE =
° (Linear pair) - Thus, ∠A = ∠
- ∠A + ∠
= ∠ + ∠C = ° (Angles on the same side of transversal) - Since ∠A = ∠B: ∠D = ∠
- Considering the triangles ΔABC and ΔBAD: AB =
(common side) - ∠ DAB = ∠
(Already proved) and AD = (Given) - ΔABC ≅ Δ
by congruency - By CPCT: AC =
- Hence, proved.
