Exercise 8.2
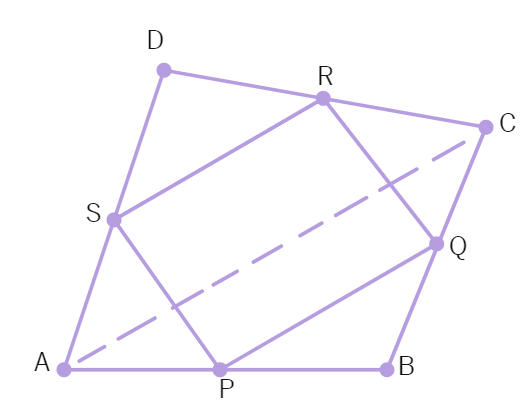
ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see Fig 8.20). AC is a diagonal. Show that :
(i) SR || AC and SR =
(ii) PQ = SR
(iii) PQRS is a parallelogram.
Solution:
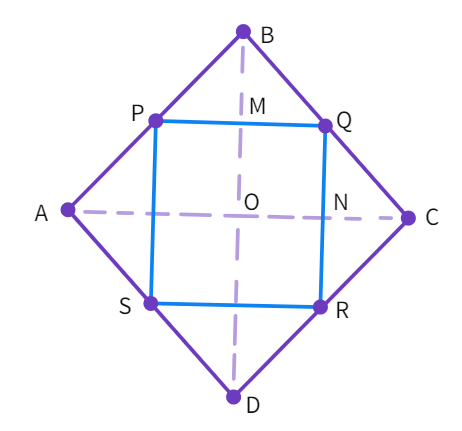
2. ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
Let's join the diagonals AC and BD.
- Considering the triangles ΔDRS and ΔBPQ:
- DS =
(Halves of the opposite sides of a rhombus) - ∠SDR = ∠
(Opposite angles of the rhombus) and DR = (Halves of the opposite sides of the rhombus) - by
congruency rule: ΔDRS ≅ Δ - By CPCT: RS =
(i) - Similarly, in ΔQCR and ΔSAP: RC =
(Halves of the opposite sides of the rhombus) , ∠RCQ = ∠ (Opposite angles of the rhombus) - and CQ =
(Halves of the opposite sides of the rhombus) - by
congruency rule: ΔQCR ≅ Δ - Therefore, RQ =
(ii) - Thus, the quadrilateral PQRS has all
sides. - Now consider the ΔCDB: we have R and Q as the mid points of CD and BC, respectively. Thus, QR ||
(i) - Similarly, in the triangle ΔADB, P and S as the mid points of AD and AB, respectively. So, we get: PS ||
(ii) - From (i) and (ii) we get, QR ||
which tells us that PQRS is a - The diagonals of the rhombus ABCD intersect each other at point O. Considering the quadrilateral OMQN: MQ ||
as PQ || AC and QN || as QR||BD. - Therefore, OMQN is a
. - We also have: ∠MQN = ∠
= ∠NOM while also knowing that the diagonals of a rhombus are to each other. - Thus, ∠NOM = ∠MQN =
° - PQRS is a
with atleast one right angle. Thus, PQRS is a - Hence, proved.
3. ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
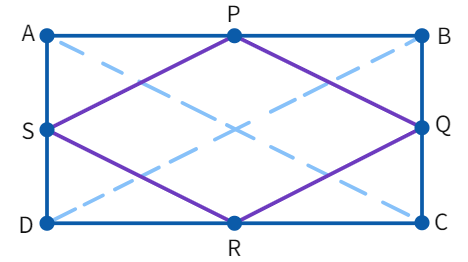
First let's join the diagonals AC and BD.
- Considering the triangle ΔABC: We have P and Q as the
of AB and BC, respectively. - Thus, with the help of the proved theorems above we know: PQ ||
and PQ = AC (i) - Similarly, in the triangle ΔADC we have: SR ||
and SR = AC (ii) - From (i) and (ii), we get: PQ ||
and PQ = - In the quadrilateral PQRS, we have one pair of equal opposite sides which are also parallel to each other, thus: PQRS is a
- Considering triangle ΔBCD, we have Q and R as mid points of side BC and CD, respectively. This gives: QR ||
and QR = BD (iii) - Similarly, for triangle ΔABD, SP ||
and SP = BD (iv) - From equations (i), (ii), (iii) and (iv) and the fact that PQRS is a
, we get: PQ = = SR = . Thus, PQRS is a . - Hence, proved.
4. ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F. Show that F is the mid-point of BC.
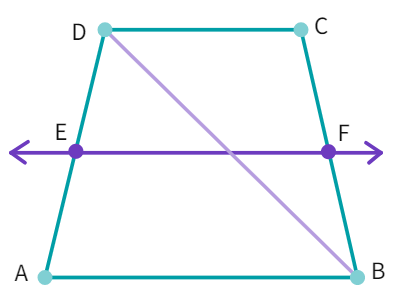
- Given that: ABCD is a trapezium in which AB || DC, BD is a
and E is the of AD. - Let BD intersected EF at G. In triangle ΔBAD, we get: E is the
of AD and also EG || . - Thus, G is the
of diagonal BD - Now in triangle ΔBDC: G is the
of BD and also GF || || . - Thus,
is the midpoint of BC - Hence, proved.
5. In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively. Show that the line segments AF and EC trisect the diagonal BD.
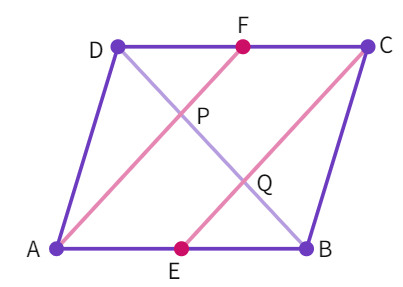
- Since ABCD is a parallelogram we have: AB||CD. So, AE||FC.
- Since AB =
we get: AB = 1/2 - AE =
as E and F are of the sides AB and CD. - Thus, AECF is a
as AE and CF are parallel and equal to each other. So, AF || - Now, in triangle ΔDQC,
is mid point of side DC and FP || (as AF||EC) - Using the converse of mid-point theorem: P is the
of DQ i.e. DP = — (i) - Similarly, in the triangle ΔAPB:
is midpoint of side AB and EQ || (as AF||EC) - Using the converse of mid-point theorem: Q is the midpoint of PB i.e. PQ =
— (ii) - From equations (i) and (i) we get: DP =
= - Thus, the line segments AF and EC trisect the diagonal BD.
- Hence, proved.
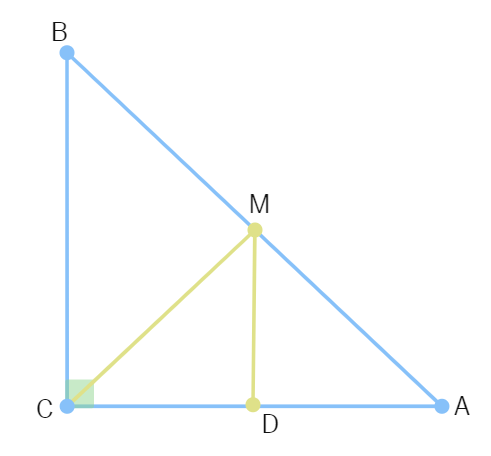
6. ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that:
(i) D is the mid-point of AC
(ii) MD ⊥ AC
(iii) CM = MA =
Solution: